Ram Pressure Stripping Made Easy

At 25 pages in length, with 30 equations and 23 figures, you could be forgiven for wondering what exactly it is about this paper that justifies the title : "Ram Pressure Stripping Made Easy". I mean, tying shoelaces is easy. Getting drunk is easy. Solving 30 equations is definitely not easy.

Well, I shall tell you. The reason is that we get periodic deliveries of catalogues from Edmund Optics. No-one seems to have the foggiest idea why we get them, but we do anyway. And one of these literary marvels featured the slogan, "Imaging Made Easy" (it was an advert for a camera). Having already rejected, "Ram Pressure Stripping For Dummies" as a title on the grounds that no editor would probably let that pass, and we'd probably be sued for trademark infringement if it did, "Ram pressure stripping made easy" was quickly adopted as an ideal substitute.

Okay, fine. So what's the paper actually about ? Yes, it's time for another, "Rhys explains a paper he worked on in the silliest way possible" post.

(I should note that I'm only the third author on this one, so as not to take credit for the first author's truly immense (many years !) amount of work on this.)


Ram Pressure Stripping : Nothing To Do With Naked Sheep

If you follow this blog much, you'll know that I spend a lot of time investigating galaxy interactions. Galaxies are pretty hefty things, so encounters between them can do a lot of damage. Stars and gas get strewn about all over the place - the "bloody entrails of galaxy interactions", as Robert Minchin once put it.

Fortunately the mess is much prettier than bloody entrails.

But the most dangerous thing to a galaxy isn't necessarily another galaxy. Under certain conditions, galaxy-galaxy interactions can be devastating. You might think that this would be when galaxies collide head-on or at terrifying speeds, so the more galaxies and the faster they move, the more dangerous things get. You'd be wrong.

It turns out the most hazardous environment for galaxies are small groups, where interactions occur most slowly. The thing is, galaxies aren't cars. The processes at work have nothing to do with tensile strength or whether the drivers made eye contact beforehand. Instead, galaxies are completely at the mercy of gravity. And what you need for gravity to do the most damage - to perturb the stars and gas most strongly and whack 'em out of their nice stable orbits - is acceleration... and all that takes is mass and time. The longer the encounter lasts, the greater the induced acceleration, and the worse the destruction. So the slower galaxies are moving, the longer and more devastating each encounter is.

Sticking with the disgusting metaphors, tidal encounters are a bit like galactic torture. The higher the force the worse things get, but what you really need is a continuous force over time.

In a galaxy cluster things are different. There there can be hundreds or thousands of galaxies, and the huge mass of the cluster means they're all moving very, very fast (typically thousands of times faster than the proverbial speeding bullet). It's certainly chaotic, but it's so chaotic that, like a movie so-bad-it's-good, it's not actually that awful for the galaxies. They have lots of encounters with other galaxies, but they're all over so blindingly fast that there's not much time for any damage to be done. And because they're all in random directions, whatever minor disturbance they do cause tends to be balanced out. Only very rarely are there any seriously damaging collisions; most of the time the encounters don't remove more than 5-10% of the gas (even after many billions of years of repeat encounters) and even less stars.

For dwarf galaxies things are a bit worse, but for massive galaxies at least, clusters are like swarms of lazy, passive bees that just want to buzz around all day and never sting anyone.

Unfortunately, clusters are more than just a giant swarm of galaxies moving at the kind of speeds normally associated with a teenager's wrist. They also contain their own gas : really hot, incredibly thin (insert offensive supermodel joke here), but filling the entire cluster. All galaxies that move through this intracluster medium (ICM) push on this gas as they move through it, exerting a ram pressure as they push it out of the way.

Some galaxies - the really crappy discount supermarket ones that nobody likes - don't have their own gas. They're not much affected by this ram pressure : the ICM can simply flow between the stars pretty much unaffected. But others do have their own gas, and that causes problems. Since it fills the whole volume of a galaxy, this gas has to interact with the ICM. If the galaxy moves slowly enough, then the ICM can basically flow around the galaxy and not cause too many problems. If it goes too fast though, then the ram pressure can become so high it can push the galaxy's gas right out into the intergalactic void where it's lost forever.

Stars take up a miniscule fraction of a galaxy's volume. So when a galaxy consisting of just stars encounters some external gas (left), it can flow right through it unimpeded. That's not possible for galaxies which do have their own gas (right) since the gas fills the entire galaxy : the external gas inevitably collides, causing a build-up of pressure.

So in clusters, galaxies have nothing much to fear from each other because they're moving so fast. Speed may save them from each other, but that's also exactly what makes the ICM so dangerous, able to strip out the entire gas content of a galaxy in a single orbit. And without gas, star formation completely shuts down. Ram pressure stripping is probably the most important mechanism driving galaxy evolution in clusters.


Let's simulate it then !

We can certainly do that, yes. We can numerically simulate galaxies falling through clusters and see how the two different gas components interact with each other. Here's one I made earlier :

This is a particularly smooth example. Usually much more turbulence
develops in the stripped gas wake.

This isn't exactly fast though. Setting up simulations like these is a laborious and time-consuming procedure to say the least. Wouldn't it be nice if we had some simple equations to tell us the main results ? Like, how much gas is gonna be lost given a certain amount of ram pressure acting for a certain time. Or what the size of the surviving gas disc would be after a particular bout of pressure, or how much gas would be displaced but eventually fall back to the galaxy due to gravity.

The very basics of this have already been done, in a classic 1972 paper by Gunn & Gott. It doesn't really say all that much though, apart from how strong the pressure needs to be and at what radius in the galaxy the gas will be stripped. That's nice, but the first author wanted more. He reckoned that you could get a lot more information from the basic equations without having to do all the horrible business of running computationally demanding simulations.

Regular readers might be expecting that I'll now begin a highly detailed breakdown of exactly what the paper says. Well for once, I won't. For one thing it's highly technical and I don't claim to understand every detail, and for another I'm trying very hard to write shorter blog posts. So I'm going to bottom line it.


What did we find ?

Famous poster image from the VIVA survey, showing that gas
discs get smaller closer to the cluster centre.

First, the effect of the ram pressure depends on how quickly it lasts. Stars in the galaxy don't just orbit around the centre, but also move up and down slightly as well. The effect of the ram pressure turns out to be quite different depending on whether the pressure "pulse" lasts for more or less time than this period of oscillation.

Second, the equations allow us to calculate the deficiency of a galaxy - how much gas it's lost in comparison to a similar undisturbed galaxy - given some basic parameters (total mass, rotation speed, that sort of thing). This makes it easy to compare with observations, since often we can only measure the mass of gas and not its radius.

Third, we can examine how much gas the ram pressure would truly strip and push off into deep intergalactic space and how much would just be temporarily displaced, only to fall back to the galaxy after a short time. Some observed streams in galaxies might not indicate true gas stripping but only this temporary displacement.

Fourth, we can make surprisingly detailed comparisons with observations. Knowing where a galaxy is in a cluster, and knowing how dense the ICM is that that point, we can predict how much ram pressure we expect it to experience at that point. And given its deficiency measurement, we can also get the pressure needed to remove that much gas. So we can work out whether we expect a galaxy to be currently stripping, not stripping, or if it was stripping in the past. And by making some assumptions about the orbit, we can even estimate for low long it's been losing gas.


That sounds neat ! Can I have a go ?

Yes you can ! If you were worried about having to do nasty things to equations, worry no more. Probably the most useful result of the paper is not the underlying equations, but the online, interactive simulations that allow anyone to use them without doing any calculations. The first author is a master of javascript (his whole webpage is well worth checking out). I am not, but I did at least persuade him that the tools he routinely developed to play with the results would be worth including in the paper.

What I really like about these is that they can be run in a few seconds (or maybe a few minutes at most), in an ordinary web browser with no installation procedure needed. Compared to other ram pressure simulations, which take hours, days or even much longer, their sheer convenience is so high it's practically erotic. They are much, much better for playing around and getting an intuitive feel for what's happening than any conventional ram pressure simulation. And though there are cases where they won't be entirely accurate and the much more complex models are needed, as long as you don't push them too far they give results which are really rather nice.

So what's on offer ? The full list of Joachim's amazing suite of javascript applets can be found here. Some highlights for ram pressure stripping enthusiasts (full instructions and descriptions can be found in the links) :

GalaxyObserved : Not really a simulation, but a way to help visualise what a stripped gas tail would look like in real observational data given a specified viewing angle

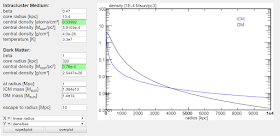
Dark matter in galaxy clusters : simple but extremely helpful little plotting tool to show the different distributions of dark matter and gas in clusters. The y-axis doesn't just have to be density - it can also be total enclosed mass, escape speed or escape time, for example. The kind of parameters you* often find yourself thinking, "hmm, that'd be nice to have, but it's hard to calculate, so oh well."

* Well I do at any rate.

Interactive ram pressure stripping : watch a galaxy get stripped in your browser ! Runs a simple test particle model with ram pressure right before your eyes. You can enter the parameters of the galaxy, the gas it encounters, how long the ram pressure pulse should last, etc. By default it plots the galaxy face-on, colouring the particles to indicate if they're still in the disc, displaced, or fully stripped. But you can use it to plot a whole bunch of other things, including what the galaxy's hydrogen spectrum would look like. Check it out ! The defaults give a nice result.

Looks complicated ? Well it is, to be honest. But it's remarkably easy to use and I guarantee it's at least 97 billion times easier than running a full hydrodynamic simulation.

So that's it : ram pressure made as easy as humanly possible. How far can we take this ? Well, in a future post I'll look at what happened when we did something rather old-fashioned : we made a prediction and tested it. Science may be more complicated than this traditional approach, but dammit it's satisfying to do once in a while. For now, let me just say that these models allow us to establish parameters for large numbers of galaxies that it just wouldn't be feasible to get using more conventional simulations. Although we must always be cautious, they point to a very interesting solution to a problem that's had me scratching my head for several years. But you'll just have to wait and see what that is.